Tree-measuring -- an Alternative to Spring

"Man is the measure of all things ...of things that are, how they are ...and of things that are not, how they are not.” (Protagoras)

This tree measures 58.4 feet in height.

It’s time for April’s Tree-following post, but there’s nothing to report as my cottonwood doesn't appear to have changed at all since a month ago.  So I decided to measure it.  “It is interesting and easy to determine the height of certain favorite trees” wrote Woodbridge Metcalf in Native Trees of the San Francisco Bay Region (1970, University of California Press).  I rounded up a crew and we headed into the field to determine the height of the cottonwood, using various methods.  It wasn’t as easy as Dr. Metcalf suggested, but he was right that it was interesting.

The day was cold, gray and windy, with sunny moments and occasional flurries of light snow.

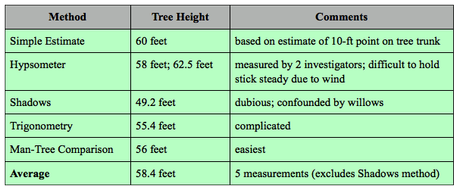
1.  Simple Estimate
Mike guessed at the point on the tree 10 feet above the ground, and estimated it took 6 of these lengths to reach the top, for a total of 60 feet.  Then the cold wind drove him away.

2.  Hypsometer
We next used a yardstick as a hypsometer (instrument for measuring height).“Measure on the ground to a distance of 25 feet from the tree.  Standing at this point, hold the ruler vertically at arms length (25 inches), with the zero point on a line from your eye to the base of the tree.  Then ‘sight’ along the ruler to the top of the tree.  Note the reading at the point where the line of sight intersects the ruler scale.  If the line of sight intersects at 20.5 inches, the tree is 20.5 feet tall.  For a taller tree, mark off a distance 50 feet from the base of the tree and double the reading on the ruler.” (Woodbridge Metcalf)

The cottonwood clearly was taller than 25 feet.  In fact we used four times the distance to get a good perspective and so had to quadruple the intercept.  My arm-length is 21 inches so I stood 84 feet from the tree.  The intercept on the yardstick was 14.5 inches, making the tree 58 feet tall (4 x 14.5).Dave tried it with his 24-inch arm-length and came up with 62.5 feet -- big difference, maybe because it was hard to keep the yardstick vertical in the wind.

3.  Shadows
“On a sunny day the height of a tree that stands on fairly level ground can be determined by relating the length of its shadow to the shadow of a staff of known height.  The staff shadow is to the height of the staff as the tree shadow is to the height of the tree.”

When the sun came out for a bit, we applied the Shadows method.  A 4-foot staff cast a 3.25-foot shadow.  Unfortunately the tree shadow ended in a stand of shrubby willows, so we tried lying on the ground among the willows to see where the tip would land.  Two of us independently estimated the shadow to be 40 feet long.

If X is the height of the tree, then 3.25 is to 4 as 40 is to X, or 4/3.25 = X/40. 

X = 160/3.25 = 49.23.  The height of the tree is 49.2 feet.

Pesky willows to right of tree caught and distorted its shadow.

4.  Trigonometry
The engineer on the crew wanted to use trigonometry.  This involved measuring the angle from level of a line from the ground to the top of the tree with an iPhone tilt-meter app, and then finding the tangent (see table) and calculating the height.  The angle was 29º and the height was 55.4 feet.

5.  Man-Tree Comparison
Finally, I photographed a man of known height standing next to the tree.  On the photo I drew and measured lines representing the height of each.  The ratio of the heights of man and tree is the same as the ratio of the lengths of the two lines.

man-line = 1.7 cm; tree-line = 16.8 cm; man-height = 5.75 ft

tree-height = (16.8/1.7) x 5.75 ft = 56 ft

Click on image and look close to see man next to tree.

Results and Discussion
The average height was 58.4 feet, not counting the Shadows results.  The Man-Tree method was the easiest, and probably the most accurate given the conditions.
So there you have it -- tree-measuring -- something to do with your favorite tree while waiting for spring.  And if you aren't yet following a tree and would like to, visit Lucy’s Loose and Leafy Tree Following page to sign up.