The problem is:
1. There are eleven islanders who all weight exactly the same and one who is either heavier or lighter than those eleven.
2. You have a see-saw with accurately marked seats that you can use as weighing scales.
3. You have to find out which islander is a different weight, and whether he/she is heavier or lighter than the others.
4. Can you always solve it in a maximum of three comparisons?
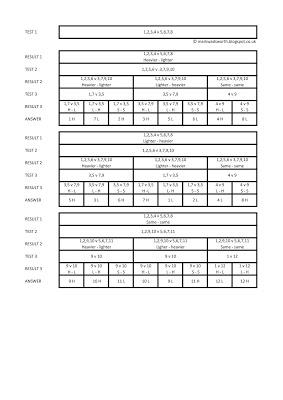
After four or five hours of trial and error, I have found that the answer is "yes" and come up with an easy to follow mechanical system (no doubt one of dozens). Pdf below (click to enlarge).
Worked example
You start by numbering the islanders 1 to 12.
Let's assume 6 is heavier, but you don't know that yet.
Test 1 is always 1,2,3,4 on one side of the see-saw against 5,6,7,8 on the other.
Result 1 is 5,6,7,8 are heavier, so go to the second table.
Test 2 is 1,2,5,6 against 3,7,9,10.
Result 2 is 1,2,5,6 are heavier.
Test 3 is 3,5 against 7,9.
Result 3 is both sides weight the same
Answer is then read off in the bottom row of the second table, "6 H"
If anybody spots a mistake, please leave a comment :-(