Bonds or Debentures: - These are debt instruments or securities. In case of abond/debenture the stream of cash flows consists of annual interest payments and repayment of principal. These flows are fixed and known.
The Value of the Bond: - It can be found by capitalising cash flows at a rate of return, which reflects their risk. The market interest rate or yield is used as the discount rate in case of bonds (or debentures). The basic formula for the bond value is as follows:
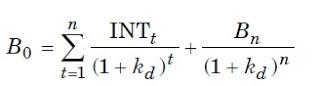
Yield to Maturity: - A bond’s yield to maturity or internal rate of return can be found by equating the present value of the bond’s cash outflows with its price in the above equation.
Zero-Interest Bonds (called zero-coupon bonds in USA) do not have explicit rate of interest. They are issued for a discounted price; their issue price is much less than the face value. Therefore, they are also called deep-discount bonds.The basic discounting principles apply in determining the value or yield of these bonds.
Preference shares have a preference over ordinary shareholders with regard to dividends. The preference dividend is specified and known. Similarly, in the case of redeemable preference share the redemption or maturity value is also known. Preference share value can be determined in the same way as the bond value. Here the discount rate will be the rate expected by the preference shareholders given their risk. This risk is more than the risk of bondholders and less than the equity shareholders.
Value of the Share (General) Cash flows of an ordinary (or equity) shareconsist of the stream of dividends and terminal price of the share. Unlike the case of a bond, cash flows of a share are not known. Thus, the risk of holding a share is higher than the risk of a bond. Consequently, equity capitalisation rate will be higher than that of a bond. The general formula for the share valuation is as follows:
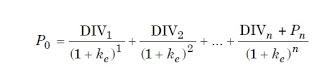
As the time horizon, n, becomes very large (say, extends to infinity) the present value of future price approaches zero. Thus the term Pn disappears from the formula, and we can use the following equation to find the value of a share today:
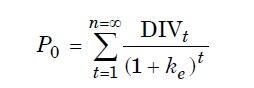
Value of the Share (Zero Growth) If dividends do not grow, then capitalisingearnings can determine the share value. Under no-growth situation, earnings per share (EPS) will be equal to dividends per share (DIV) and the present value is obtained by capitalizing earnings per share:
Value of the Share (Constant Growth) In practice, dividends do grow over years. If we assume dividends to grow at a constant rate, g, then DIV1 = DIV0 (1 + g), DIV2 = DIV1 (1 + g), DIV3 = DIV2 (1 + g)..., and the share price formula can be written as follows: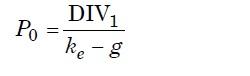
This formula is useful in calculating the equity capitalisation rate (ke) when the price of the share (P0) is known.Under the assumption of constant growth, the share value is equal to the capitalized value of earnings plus the value of growth opportunities as shownbelow:
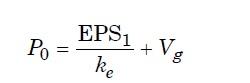Value of Growth Opportunities (Vg) Given a firm’s EPS, ROE, the equity capitalisation rate, retention ratio and constant growth, the growth opportunities can be valued as follows:
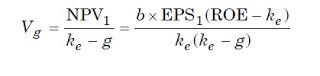E/P Ratio and Ke Relationship between the earnings-price ratio and capitalisation rate as follows:
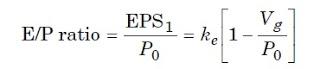The E/P ratio will equal the capitalisation rate only when growth opportunities are zero, otherwise it will either overestimate or underestimate the capitalisation rate.
