Differences Between Rational and Irrational Numbers

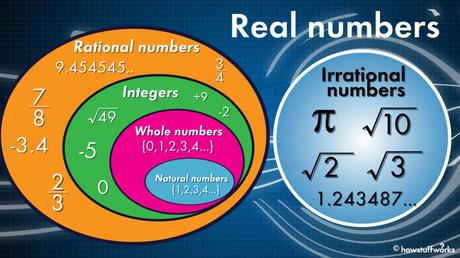
When you hear the words "rational" and "irrational," they might bring to mind the ruthlessly analytical Spock in "Star Trek." However, if you're a mathematician, you probably think about ratios between integers and square roots.

In the field of mathematics, where words sometimes have specific meanings that are very different from everyday usage, the difference between rational and rational irrational numbers has nothing to do with emotions. Since there are an infinite number of irrational numbers, you would do well to get a basic understanding of them.

Properties of irrational numbers

"When remembering the difference between rational and irrational numbers, think of one word: ratio," explains Eric D. Kolaczyk. He is a professor of mathematics and statistics at Boston University and director of the university's Rafik B. Hariri Institute for Computing and Computational Science & Engineering.

"If you can write a number as a ratio of two integers (for example, 1 divided by 10, -5 divided by 23, 1,543 divided by 10, etc.), then we put it in the category of rational numbers," says Kolaczyk in an email. "Otherwise we say it's irrational."

You can express a whole number or a fraction (divisions of whole numbers) as a ratio, using a whole number called a numerator on top of another whole number called a denominator. You divide the denominator by the numerator. That might give you a number like 1/4 or 500/10 (also known as 50).

Irrational numbers: examples and exceptions

Irrational numbers, unlike rational numbers, are quite complicated. As Wolfram MathWorld explains, they can't be expressed as fractions, and if you try to write them as a number with a decimal point, the digits just keep going, without ever stopping or repeating a pattern.

So what kind of numbers behave in such a crazy way? In short, those who describe complicated things.

Pi

Perhaps the best-known irrational number is pi - sometimes written as π, the Greek letter for 'p' - which expresses the ratio between the circumference of a circle and its diameter. As mathematician Steven Bogart explained in this 1999 Scientific American article, that ratio will always equal pi, regardless of the size of the circle.

The story continues

Ever since Babylonian mathematicians tried to calculate pi nearly 4,000 years ago, successive generations of mathematicians have continued to plug away and come up with longer and longer sequences of the decimal expansion with non-repeating patterns.

In 2019, Google researcher Emma Hakura Iwao managed to expand pi to 31,415,926,535,897 digits.

Some (but not all) square roots

Sometimes a square root (that is, a factor of a number that, when multiplied by itself, gives the number you started with) is an irrational number unless it is a perfect square that is an integer, such as 4, the square root of 16.

One of the most notable examples is the square root of 2, which is 1.414 plus an endless string of non-repeating digits. That value corresponds to the length of the diagonal within a square, as first described by the ancient Greeks in the Pythagorean theorem.

Why do we use the words 'rational' and 'irrational'?

"Indeed, we usually use 'rational' to mean something more based on reason or something like that," says Kolaczyk. 'Its use in mathematics appears to have appeared in British sources as early as the 13th century (according to the Oxford English Dictionary). If you trace both 'rational' and 'ratio' back to their Latin roots, you see that in both cases the root is about 'reasoning', broadly speaking."

What is clearer is that both irrational and rational numbers have played an important role in the progress of civilization.

Although language likely dates back to the origins of the human species, numbers came much later, explains Mark Zegarelli, a math teacher and author who has written ten books in the "For Dummies" series. Hunter-gatherers, he says, probably didn't need much numerical precision, other than the ability to roughly estimate and compare quantities.

"They needed concepts like, 'We're out of apples,'" Zegarelli says. "They didn't need to know, 'We have exactly 152 apples.'"

But when people started carving out plots of land to build farms, build cities, and produce and trade goods, traveling further away from their homes, they needed more complex math.

"Suppose you build a house with a roof whose height is as long as the slope from the base at the highest point," says Kolaczyk. "How long is the length of the roof surface itself, from the top to the outer edge? Always a factor of the square root of 2 of the rise (run). And that is also an irrational number."

The role of irrational numbers in modern society

In the technologically advanced 21st century, irrational numbers continue to play a crucial role, according to Carrie Manore. She is a scientist and mathematician in the Information Systems and Modeling Group at Los Alamos National Laboratory.

"Pi is an obvious first irrational number to talk about," Manore says via email. "We need it to determine the area and circumference of circles. It is critical for calculating angles, and angles are critical for navigation, building, surveying, engineering and more. Radio frequency communication relies on sinuses and cosines involving pi."

Furthermore, irrational numbers play a key role in the complex mathematics that power high-frequency stock trading, modeling, forecasting, and most statistical analysis - all activities that keep our society running.

"In fact," Manore adds, "in our modern world it almost makes sense to ask instead, 'Where are irrational numbers? not being used?'"

This article was updated using AI technology, then fact-checked and edited by a HowStuffWorks editor.

That is interesting

Computationally, "we almost always use approximations of these irrational numbers to solve problems," Manore explains. "These approaches are rational because computers can only calculate with a certain accuracy. Although the concept of irrational numbers is ubiquitous in science and engineering, one could argue that in practice we never actually use a truly irrational number."

Original article: Differences between rational and irrational numbers

Copyright © 2023 HowStuffWorks, a division of InfoSpace Holdings, LLC, a System1 company