
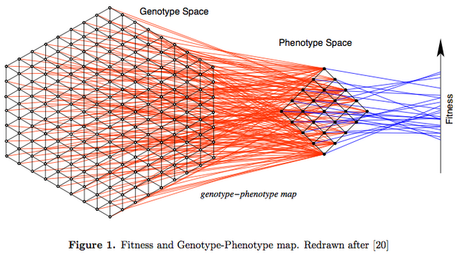
I love it. But just before this figure he has this equation:

However, I reiterate (and is why I'm reading the chapter) that this figure of a genotype-phenotype-fitness map is super cool.There are many more different genotypes (the genetic make-up of an organism) than there are different phenotypes (the combined physical attributes of the organism). This must be so, because we now know that each trait is affected by many genes; it takes more than one gene to make a trait (there may be exceptions where only one gene encodes a trait).
The figure is a conceptual map, but real g-p mapping is sort of the holy grail in evolutionary biology at the moment. With a real map like in hand evolutionary dynamics can be predicted, and we will be able to say which genetic changes are required to change the phenotype. However, realistically we can only map a very small portion of the genotype on to the phenotype, and there even seems to be some confusion about what the proper answer is to the question of what the genotype-phenotype map looks like. Hopefully the answer won't be too mathy...
References
[1] Peter F. Stadler and Christopher R. Stephens (2003). Landscapes and Effective Fitness Comm. Theor. Biol, submitted to, 2003. Santa Fe Insitute Working Paper: 0210048. DOI: 10.1080/08948550302439
[20] A testable genotype-phenotype map: Modeling evolution of RNA molecules. In: Lässig, M. and Valleriani, A., editors, Biological Evolution and Statistical Physics, pp. 56–83. Springer-Verlag, Berlin, 2002.


